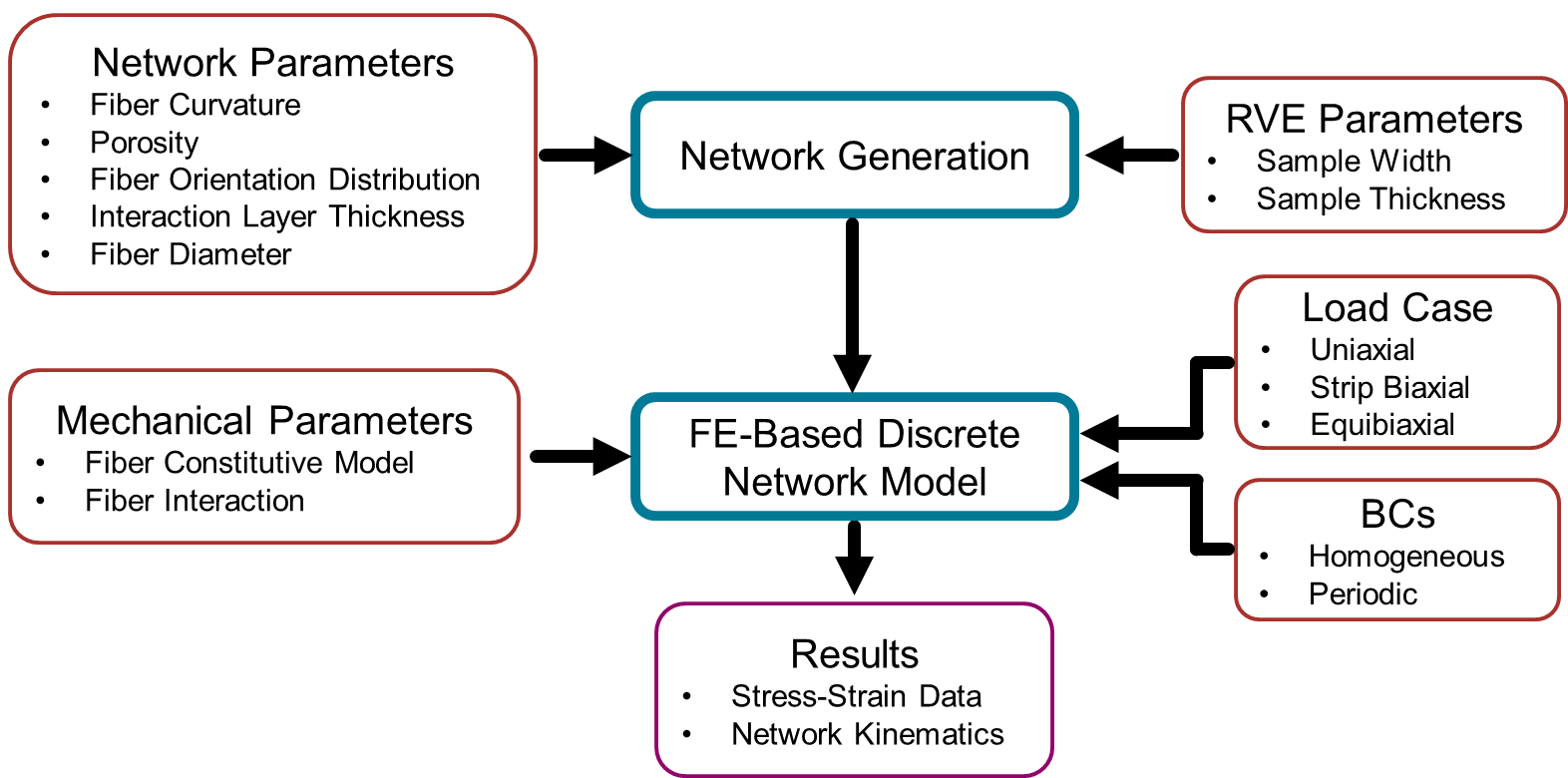
Tissue Scaffolds for Intervertebral Disc Repair
Electrospun Networks as Tissue Engineered Scaffold
Electrospinning is well suited for the manufacturing of tissue engineered scaffolds, due to its capability to create planar polymer fiber networks with widely tunable topology and properties. By control of the process different network parameters can be influenced, such as fiber diameter, curvature and orientation, fiber and cross-link density and porosity. This manufacturing process allows therefore to explore a wide design space for the scaffolds.
The present project focus on tissue engineering scaffolds for the repair of the annulus fibrosus, the outer ring of the intervertebral disc.
Among the different requirements to those scaffolds, there is the need to satisfy the criteria of mechanical biocompatibility which for fiber networks is reflected in the load transfer and deformation mechanisms across different length scales. Our goal is the creation of numerical models able to predict the mechanical behaviors of the networks affecting its mechanical biocompatibility on the different length scales based on the network architecture parameters. This would allow to virtually explore the huge design space of the electrospun network and select the most promising candidates for further investigations.
Discrete Network Model for Electrospun Networks
To investigate the macroscopic response of such networks to externally applied loads and to analyze the transfer of stress and strain over the different length-scales, a numerical model has been developed using a discrete network approach.
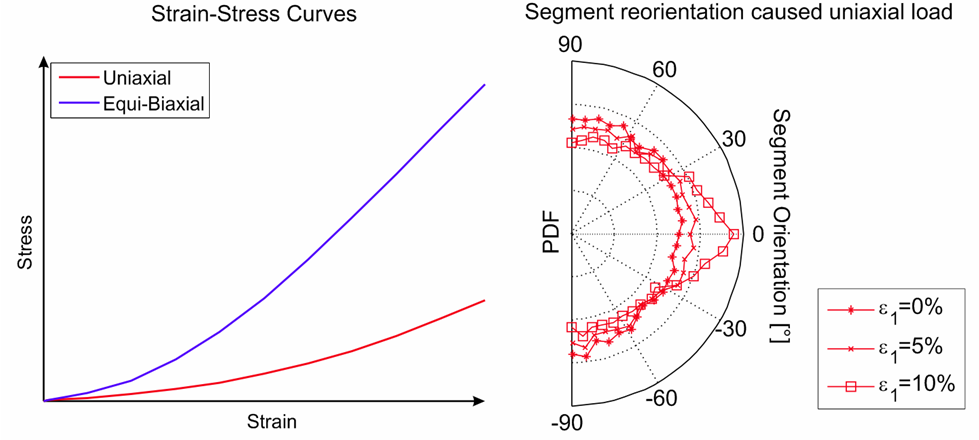
The developed network model allows to relate numerically the effect of the major network parameters on the mechanical response. Of interest are is the global mechanical behavior given by the strain-stress curves and deformation kinematics, as also parameter defining the deformation mechanism at microscopic scale. Examples for the latter are the deformation induced fiber reorientation (as shown in the next figure) and the degree of non-affinity of the strain field within the network.
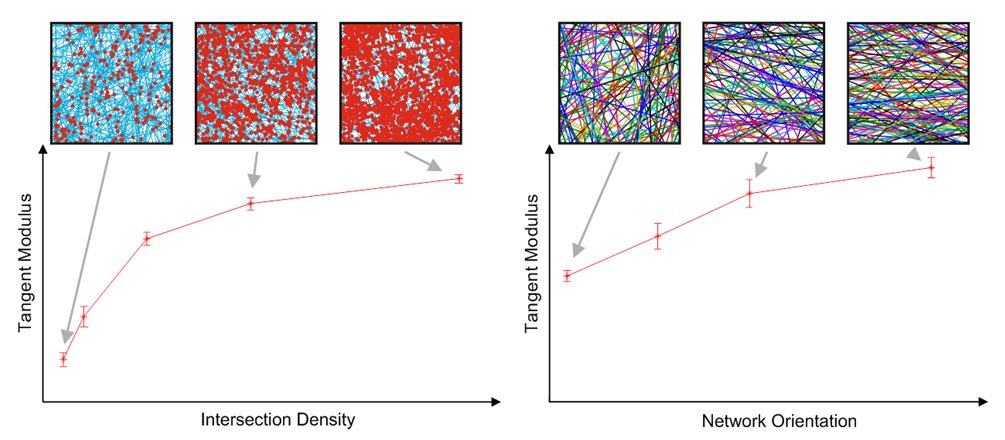
The following figures show how the network stiffness can be influenced through the cross link density or the alignment of the fibers.
Project Lead
Manuel Zündel